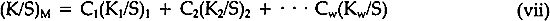THE KINETICS OF FADING: OPAQUE PAINT FILMS PIGMENTED WITH ALIZARIN LAKE AND TITANIUM DIOXIDERuth Johnston-Feller, Robert L. Feller, Catherine W. Bailie, & Mary Curran
5 DISCUSSIONTHE FORMATION OF a yellow intermediate compound found in these studies contrasts with the results obtained previously with glazes (alizarin lake, without titanium dioxide, applied at incomplete hiding).10 In that investigation we found that the color of the dark samples also made an abrupt shift towards yellow during fading. In the case of the dark glazes, however, the shift towards the yellow was calculable on the basis of the changes taking place in the spectrophotometric curve shape as the concentration of alizarin diminished; no yellow compound was ever needed to match the color of the faded glazes. Included in our investigations were a number of samples of alizarin lake mixed with titanium dioxide at incomplete hiding, not reported in the paper on glazes nor in this In the publication on the glazes, we described a simplified version of the Kubelka-Munk equation for incomplete hiding, a “single-constant” equation, useful but not as accurate as the two-constant equation in which both K and S are variables. The scattering coefficient, S, was assumed to be constant, and only the absorption coefficient, K, was assumed to vary during the exposure. We can also describe a simplified equation applicable to a relatively transparent pigment, such as alizarin lake, in mixture with a highly scattering white pigment in a paint film applied at complete hiding. Again the assumption is made that the scattering of the paint film is constant. As in the case of the glazes, we may then assume that the only change in color is caused by a change in the absorption coefficient, K. On this basis we can calculate a color match to the faded samples using a simplified “single-constant” equation applicable to the case of complete hiding:
If a pigment fades directly to a colorless form—that is, if no intermediate compound is formed which absorbs light of the same wavelength as that absorbed at the absorption maximum of the pigment being tested—a further simplification can be made. If both assumptions mentioned are true—S does not change, and K at the absorption maximum is not affected by the formation of another species absorbing at this wavelength—then we can apply kinetic analysis by using the (K/S) value (Equation iv) at the wavelength of maximum absorption as the measure of concentration of the absorbing pigment remaining. Thus, to analyze the kinetics of the fading process, the log (K/S) at the absorption maximum can be plotted against time or net exposure. In two of the publications cited5,6 the authors used this abbreviated method to demonstrate the first-order behavior of the colorants. |